AT 309 Week 10: Introduction into Coordinate Systems by ESRI

 Introduction

Continuing with the ArcGIS theme, the week 10 lab was centered around an ESRI course about coordinate systems. The course utilizes ArcGIS Pro to teach users about the importance of understanding coordinate systems. 

Spheroid

Earth is a spheroid that is slightly wider at its equator. Many spheroids have been made over time to represent Earth. More accurate spheroids are made as better technology develops. The spheroids used in this course are Clark 1866, International 1924, and WGS 1984. 

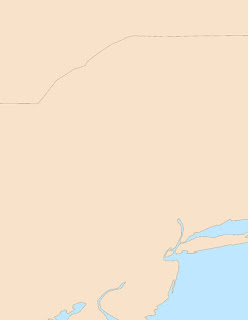Base map of Long Island

The project in ArcGIS Pro starts with a base map of the world. 

WGS 1984 Layer

The first layer added was WGS 1984. Comparing this projection to the base map, it is easy to see that WGS 1984 added more detail along the borders. 

All 3 projections applied at once.

At first glance there does not seem to be much difference in the three coordinate systems. They are all about the same shape and provide the same amount of detail.

Zoomed in view of the layers. 

The layers are uniform in shape and size, but they are all in different locations. The measure tool in ArcGIS Pro is useful here because it allows users to see measure how far apart the layers are. The distance between Clarke 1866 (purple) and WGS 1984 (orange) is around 246 meters. The distance between International 1924 (green) and WGS 1984 is about 93 meters. 

Adding Coordinate Systems to Points

The second part of the course involved plotting points on a map. There were two sets of points: one with a coordinate system and one without a coordinate system. 

Points (with WGS 1984) added.

The points represent African cities. Both sets of points represent the same cities so users can see the importance of coordinate systems. The points in the image shown above already had a coordinate system so they showed up in the correct position. 

Points (without coordinate system) added.

Map of points (without coordinate system) zoomed out.

The second set of points are the same distance and shape as the first set of points, but they are scaled down too small and in the wrong location. This is because these points do not have a coordinate system to project off of. Further investigation shows that the second set of points are projected by distance (in meters) from the origin. This issue can be solved with the "Define Projection" tool in ArcGIS Pro. 

Both sets of points projected with WGS 1984.

Using this tool to apply WGS 1984 moved the points to the correct location. This shows the importance of coordinate systems. Coordinate systems use 4 components to maintain accuracy in GIS software: angular units of measure, a prime meridian, a spheroid, and a datum. When layers are added to a map, all layers need to use the same coordinate system. Data will not show up properly if coordinate systems do not match. This leads to inaccurate data that cannot be used. This is an easy problem to fix if users know about coordinate systems. That is why people working in geospatial fields need to know this.

Projections

The last part of the course focused on projections. Projections are 2D maps projected onto 3D spheroids. There are multiple types of projections and each one comes with distortion. Because distortion is inevitable, different projections are used to preserve different map properties.

Projection Name	What does it preserve?	What gets distortion?
Conformal Projection	Shape	Area
Equal Area Projection	Area	Shape
Equidistant Projection	Distance From 1 or 2 Points to Every Other Point	Shape and area.
Azimuthal Projection	Direction From 1 or 2 Points to Every Other Point	Shape and Area.
Gnomonic Projection	The Shortest Route (Distance and Direction)	Area
Compromise Projections	None	Compromise maps have some distortion on shape and area, but no extreme distortion on either.

The table above lists the types of projections, the properties they preserve, and the properties that become distorted. Different maps have different goals in terms of what they are trying to show viewers. For example, an equidistant projection will have accurate distance from one point to all other points, but distance from other points to other points will not be accurate. 

The projection activity for this part of the course showed users how to make a projection of Alaska. 

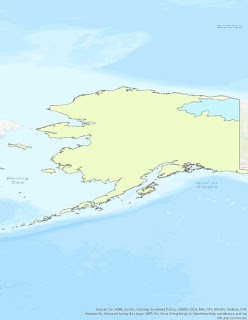Map of Alaska in WGS 1984

The image above is the Alaska layer without a projection. This was changed to an equal area conic map that will preserve area and shape.

Equal Area Conic Map of Alaska

Now Alaska looks less stretched out and distorted. Visual data is good in mapping but geospatial analysis often requires more than just accurate visual representation. Changing coordinate systems projects the data for display purposes, but measurement values stay the same. This means the data cannot be analyzed. 

The "Project" tool, in ArcGIS Pro, is useful here. This tool projects the data so measurement values are accurate. This is another important part of geospatial analysis. Once again, if users do not know to do this, the data will be inaccurate and unusable.

Conclusion

As we start to move towards data analysis in UAS at Purdue, we need to know how to ensure the data is accurate. Courses like this one teach valuable lessons about how to preserve accuracy. The course also shows the many tools ArcGIS Pro has to offer in translating data to make it appear the way we want it to. Jobs involving UAS require much more than just taking pictures. Operators need to know how collect data, but they also need to know how to use that data in GIS software.

Certificate showing completion of "Introduction to Coordinate Systems" ESRI course.